Nikola Tesla Books
Colorado Springs
June 30, 1899
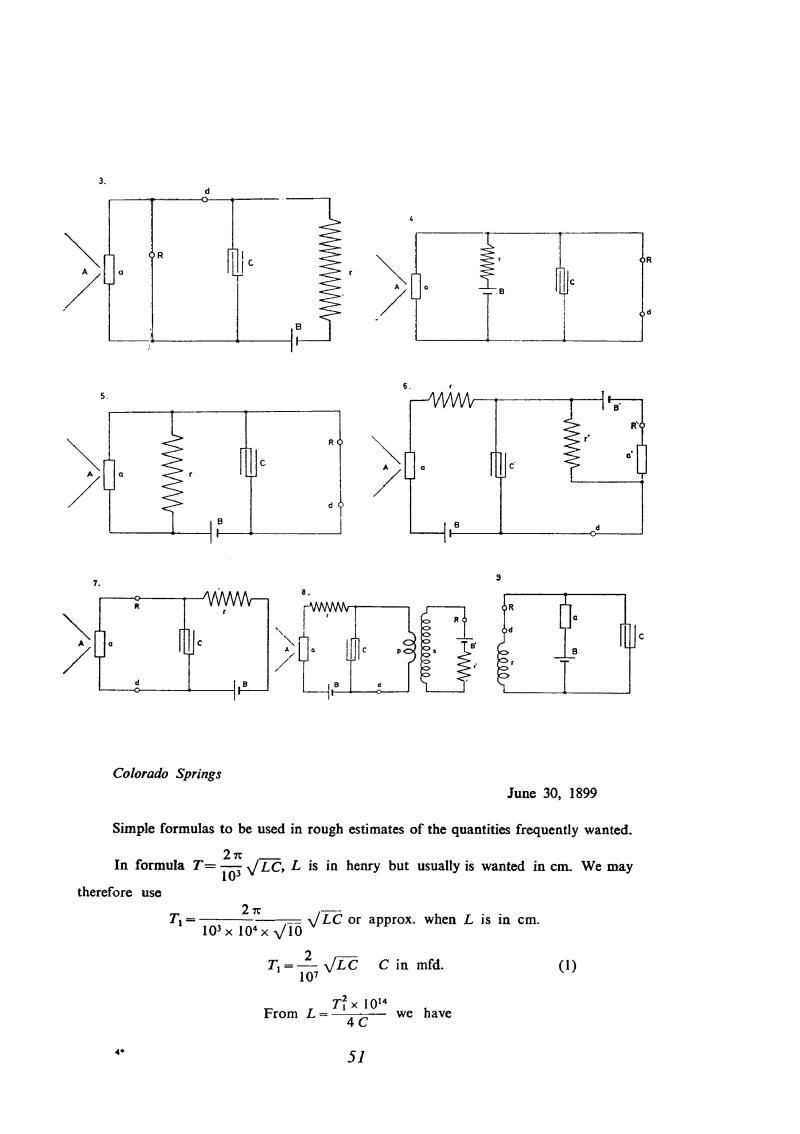
Simple formulas to be used in rough estimates of the quantities frequently wanted.
In formula T = $! { {2 \pi \over 10^{3}} {\sqrt{LC}} } $!, L is in henry but usually is wanted in cm. We may therefore use
T1 = $! { {2 \pi \over {10^{3} \times 10^{4} \times \sqrt{10}}} \sqrt{LC} } $! or approx. when L is in cm.
T1 = $! { {2 \over 10^{7}} \sqrt{LC} } $! C in mfd. (1)
From L = $! { {T_{1}^{2} \times 10^{14}} \over 4 \, C } $! we have
4*
51
Tesla: âExperiments with alternate currents of very high frequency and their application to methods of artificial illuminationâ, a lecture delivered before the AIEE, May 20, 1891, L-15.
June 29
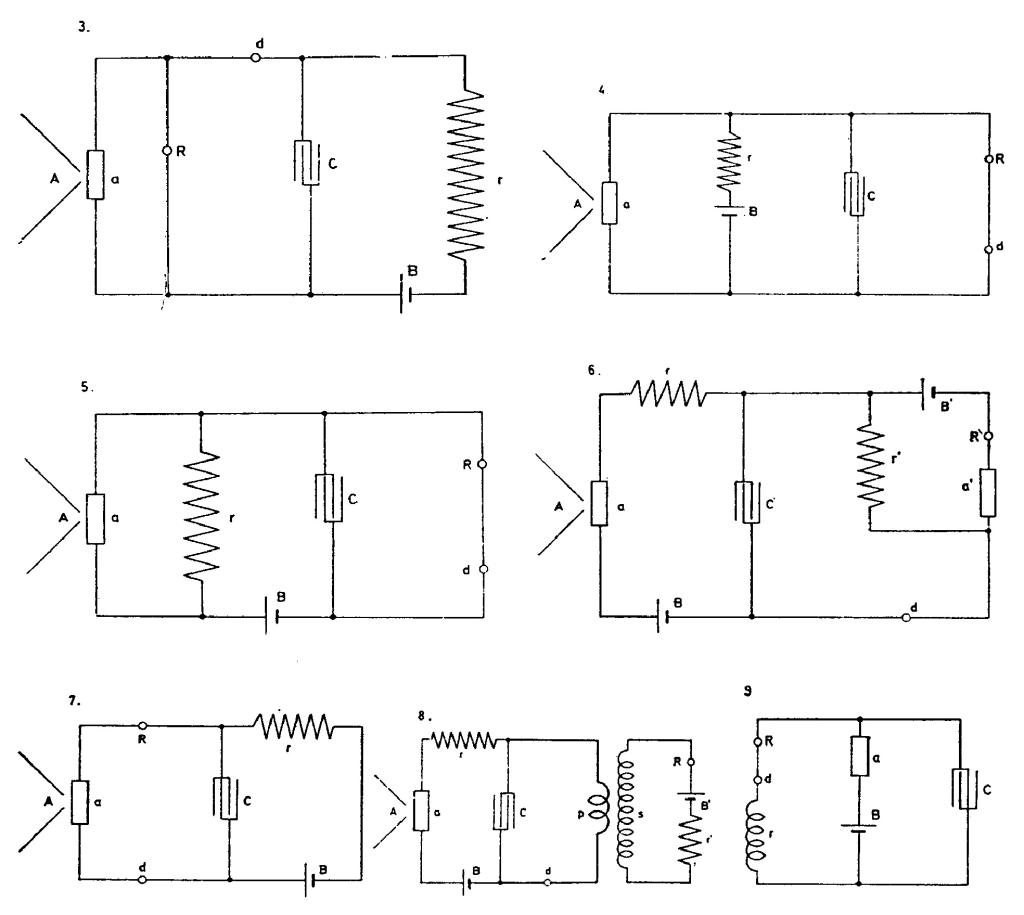
He didn't use yesterday's calculation, because he continued with a secondary with 36 turns. First detailed tests of oscillator operation did not provide expected results and Tesla attempts to determine the reasons by calculation. He gives secondary inductance, calculates the "additional coil" inductance but he doesn't use these results; then he determines free secondary vibration from the condition that total secondary wire length is equal to one quarter of wavelength. As he is already aware that the capacitances in the secondary circuit change this condition on secondary wirelength, he says in the calculation that the capacitances are neglected. With an approximate value for secondary resonant frequency he determines the required capacitance in primary circuit.
As he used small capacitances in the experiment, he concludes that he operated with high harmonics and that was the reason why he didn't achieve success.
The next day he gave several schematics of receivers with which he experimented probably for the purpose of patent submission preparation. Leonard E. Curtis is the person who appears several times pertaining to Tesla's patents as witness (please see e.g. (8,10)), or as lawyer on several patents starting in April, 1896.
June 30
Description of electric circuits in terms of mechanical analogies was at one time very popular. The resonance of an electrical circuit was likened to the swinging of a pendulum, and coupled resonant circuits to two pendulums linked together(39). Maxwell and his followers even tried for a long time to describe the electromagnetic field in terms of a mechanical model(40). Tesla's comparison of his âadditional coilâ to a pendulum is not precisely formulated but rather intuitive. He correctly discriminates between the excitation (initial conditions) and the Q-factor. He does not fully explain how he imagined that the vibrations of the three systems, the primary, the secondary and the âcombined systemâ, would be the same. By âfreeingâ the additional coil he means a weakening of the coupling between it and the secondary exciting it. He obviously had a clear understanding that a circuit can oscillate at its own resonant frequency if the coupling with an excitation circuit is loose.
June 30
Since Tesla very frequently used Thompson equation with LC circuit without losses, he found it helpful to prepare suitable equations on the basis of which with minimal calculations he can obtain desirable values.
In continuation of the notes he sorts out the impressions and results (maybe because that is the end of the month?). Comparison of events in electrical circuits with mechanical analogous systems was in the past popular. The resonance of the electrical circuit connected with pendulum, the event in linked resonance circuits with two pendulums which are mutually linked(39).
Even Maxwell and his followers attempted for a long time to describe the electromagnetic field by means of a mechanical model(40). Tesla's comparison of "additional coil" with pendulum was not precisely shaped - it is more by intuition. Tesla correctly segregated the excitation (initial conditions) and quality factor. He didn't thoroughly explain how he imagined that free vibrations of three systems would be the same: primary, secondary and "combined system". When he talks about "releasing" of additional coil, he foresees the weakening of its link with the secondary which excites it. Tesla had a clear imagination that the circuit tends to vibrate on its own resonant frequency if poorly linked with the excitation. It is strange that he didn't apply this logic on the secondary circuit which he intentionally designed to be in strong link with the oscillator primary circuit.