Nikola Tesla Books
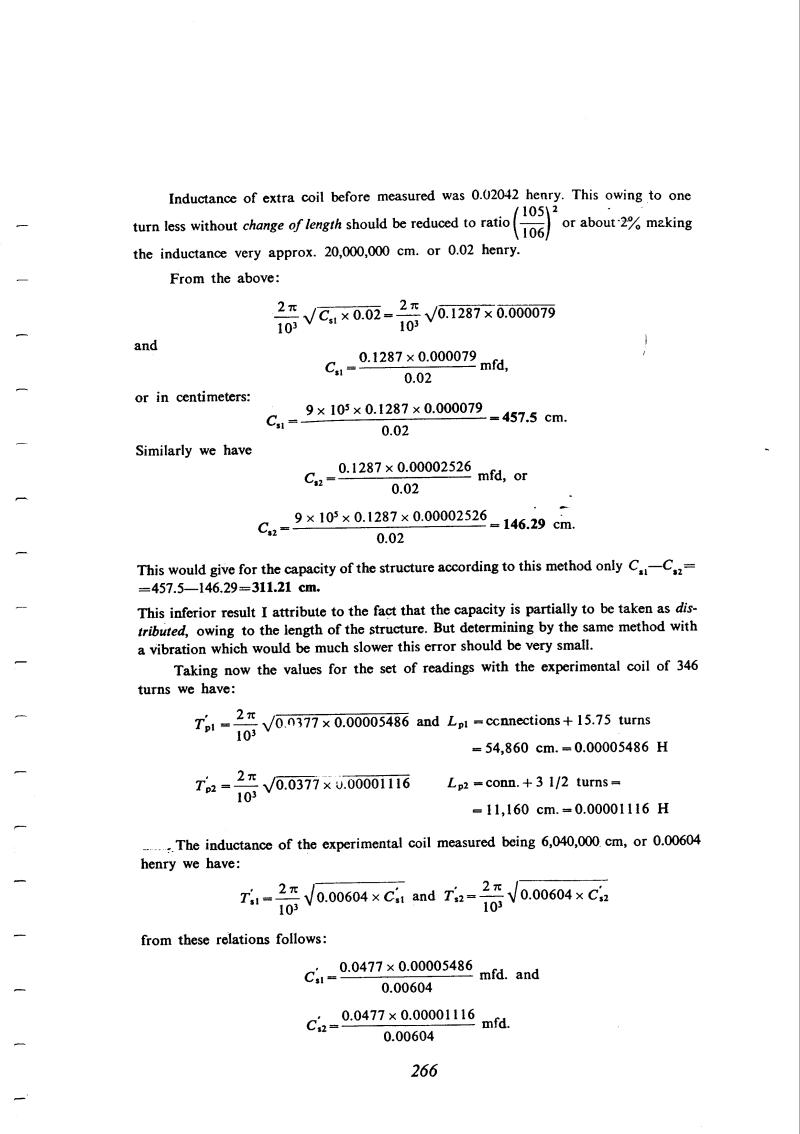
Inductance of extra coil before measured was 0.02042 henry. This owing to one turn less without change of length should be reduced to ratio $! {\left({105 \over 106}\right)^{2}} $! or about 2% making the inductance very approx. 20,000,000 cm. or 0.02 henry.
From the above:
$! {{{2 \pi \over 10^{3}} \sqrt{{C_{s}}_{1} \times 0.02}} = {{2 \pi \over 10^{3}} \sqrt{0.1287 \times 0.000079}}} $!
and
Cs1 = $! {{0.1287 \times 0.000079} \over 0.02} $! mfd,
or in centimeters:
Cs1 = $! {{9 \times 10^{5} \times 0.1287 \times 0.000079} \over 0.02} $! = 457.5 cm.
Similarly we have
Cs2 = $! {{0.1287 \times 0.00002526} \over 0.02} $! mfd, or
Cs2 = $! {{9 \times 10^{5} \times 0.1287 \times 0.00002526} \over 0.02} $! = 146.29 cm.
This would give for the capacity of the structure according to this method only Cs1 - Cs2 = 457.5 - 146.29 = 311.21 cm.
This inferior result I attribute to the fact that the capacity is partially to be taken as distributed, owing to the length of the structure. But determining by the same method with a vibration which would be much slower this error should be very small.
Taking now the values for the set of readings with the experimental coil of 346 turns we have:
| T'p1 = $! {{2 \pi 10^{3}} \sqrt{0.0377 \times 0.00005486}} $! | and | Lp1 | = connections + 15.75 turns |
| = 54,860 cm. = 0.00005486 H | |||
| T'p2 = $! {{2 \pi 10^{3}} \sqrt{0.0377 \times 0.00001116}} $! | Lp2 | = connections + 3 1/2 turns = | |
| = 11,160 cm. = 0.00001116 H |
The inductance of the experimental coil measured being 6,040,000 cm, or 0.00604 henry we have:
T's1 = $! {{{2 \pi \over 10^{3}} \sqrt{{0.00604 \times C'_{s}}_{1}}}} $! and T's2 = $! {{{2 \pi \over 10^{3}} \sqrt{{0.00604 \times C'_{s}}_{2}}}} $!
from these relations follows:
C's1 = $! {{0.0477 \times 0.00005486} \over 0.00604} $! mfd. and
C's2 = $! {{0.0477 \times 0.00001116} \over 0.00604} $! mfd
266
November 7
Measurement of the capacity of the structure at two frequencies was intended to demonstrate the reduction of effective capacity with increasing frequency. Tesla did in fact obtain a small difference, but it is dubious proof considering the accuracy of the measurements. The frequency difference was quite large, from 50 kHz to nearly 250 kHz (using âextraâ and âexperimentalâ coils).
November 7
He measures the structure capacitance with two coils, one is a new "additional coil" (please see Oct. 31). The results are similar to those of Nov. 6. An attempt to measure the structure capacitance at two frequencies was intended to prove that the capacitance is reduced when the frequency is increased. Tesla obtained a small difference but without that it is a reliable proof when we take in account the measurement accuracy. Otherwise the frequency changed substantially; from approximately 50 Hz to almost 250 KHz{during the period of obtaining the measured results with the "additional" and "experimental" coil).