Nikola Tesla Books
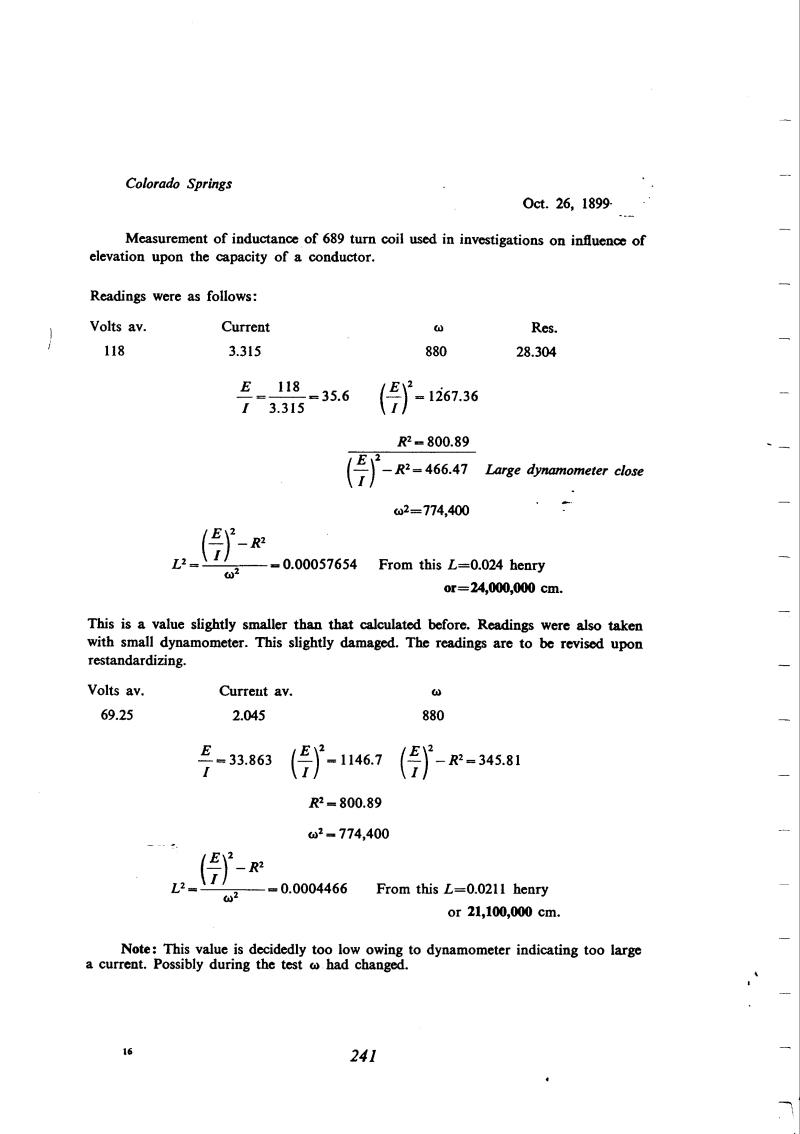
Colorado Springs
Oct. 26, 1899
Measurement of inductance of 689 turn coil used in investigations on influence of elevation upon the capacity of a conductor.
Readings were as follows:
| Volts av. | Current | Ï | Res. |
|---|---|---|---|
| 118 | 3.315 | 880 | 28.304 |
$! {{E \over I} = {118 \over 3.315} = 35.6} $! $! {\left(E \over I\right)^{2} = 1267.36} $!
$! {{R^{2} = 800.89} \over {\left(E \over I\right)^{2} - R^{2} = 466.47}} $! Large dynamometer close
Ï2 = 774,400
$! {L^{2} = {{\left(E \over I\right)^{2} - R^{2}} \over Ï^{2}} = 0.00057654} $! From this L = 0.024 henry or = 24,000,000 cm.
This is a value slightly smaller than that calculated before. Readings were also taken with small dynamometer. This slightly damaged. The readings are to be revised upon restandardizing.
| Volts av. | Current av. | Ï |
|---|---|---|
| 69.25 | 2.045 | 880 |
$! {{E \over I} = 33.863} $! $! {\left(E \over I\right)^{2} = 1146.7} $! $! {\left(E \over I\right)^{2} - R^{2} = 345.81} $!
R2 = 800.89
Ï2 = 777,400
$! {L^{2} = {{\left(E \over I\right)^{2} - R^{2}} \over Ï^{2}} = 0.0004466} $! From this L = 0.0211 henry or 21,100,000 cm.
Note: This value is decidedly too low owing to dynamometer indicating too large a current. Possibly during the test Ï had changed.
241
Tesla: âOn electricityâ, EL Rev. Jan. 27, 1897, A-101.
October 26
Tesla had already been using the 689-turn coil for several days in experiments to determine change of capacity with height of a ball. On October 18th he calculated its inductance using the formula for an infinitely long coil. Now he determines it by measuring the current and voltage at a frequency of about 140 Hz, knowing the resistance. He gives the results of two sets of measurements. He is convinced that the second set, for which he used a small dynamometer, gave low values, and this was probably so. The first set gave an inductance slightly less than calculated, but a correction of the theoretical value for the finite D/l ratio* gives a value about 6% less than that measured. Thus the calculated value ought to have been 0.023 H, while the experimental result was 0.024 H. The accuracy of the measurement method cannot now be verified but in view of the small difference between reactance and resistance it is doubtful whether it could be of the order of a few percent.
October 26
Tesla already used the coil with 689 turns for several days when experimenting in order to determine the variation of the sphere capacitance with elevation variation. On October 18 he calculated the inductance of this coil by the equation for an infinitely long coil. Now he determined this inductance on the basis of voltage and current measurement at a frequency of approximately 140 Hz and the known resistance. Tesla gives the results of two measurements groups. For the second group where he mentions a small dynamometer, he is decisive in the opinion that the obtained value is smaller than actual and that it is probably correct. The first measurement provided the inductance which is somewhat smaller than calculated. However, the corrected calculated value is approximately 6% smaller than that found when the final ratio correction d/l is taken into account*. Therefore it shows that the calculated value is 0.023 H, and the measured value is 0.024 H.
The check on the measurement method accuracy we cannot perform, but based on the small difference in the value of the impedance and the resistance we doubt that the error is more than 1%.
* Per Russell the coil inductance for very low frequency is given with(57): L = (ÏDn)2l {l + 0.424(D/l) + 0.215(D/l)2 + 0.156(D/l)4} When the reduction is done ÏD2 = 4. 5 (D is mean coil diameter), n = N/l (n is the number of turns per cm), the first member in the above equation which Tesla uses, l is the coil length. When all units are in cm the inductance L is in cm as well.